A company that is going to invest money into a new project would certainly want to know the viability or profitability of that project. To appraise the potential performance of an investment project, companies mostly use four techniques – payback method, accounting rate of return method, net present value method and internal rate of return method. Each of these four techniques evaluates project performance from a different angle and companies mostly use more than one technique to assess their forth coming projects.
Net present value (NPV) and internal rate of return (IRR) are extensively used measures to appraise investment projects. Unlike simple payback method and accounting rate of return method, NPV and IRR both take into account the time value of money which make them more reliable and practical investment appraisal techniques for companies. This article defines and explains the difference between NPV and IRR.
Definitions and meanings:
NPV:
A project’s net present value (NPV) is defined as the difference between present value of total cash inflow and the present value of total cash outflow over the life of the project.
Present value of a cash flow means the amount of cash due to be received or paid at a future point of time, discounted using an appropriate discount factor which in most cases is the company’s cost of capital.
The cost of capital of a business is the minimum combined rate of return that its investors (shareholders and creditors) expect from a business.
The projects with positive NPV promise a positive overall cash flow. Such projects are considered desirable and are therefore accepted. The project with a negative NPV indicate a negative overall cash flow. These projects are considered undesirable and are not undertaken.
IRR:
The internal rate of return (IRR) is the rate of interest at which the NPV of a project becomes zero. It means that at this interest rate a particular project stands at break-even. The projects whose IRR is greater than the company’s cost of capital are considered desirable and therefore accepted. Any project whose IRR is below the cost of capital of the company is not desirable and should be avoided.
As stated earlier that IRR is a rate at which NPV of a project equals zero, therefore any project that promises an IRR below the cost of capital indicates that its NPV is negative and it should not be undertaken.
Formula of NPV and IRR:

Let’s explain the computation of NPV and IRR with help of an example:
Example:
Julia Private Limited is a chair making company. It has an investment opportunity to invest in a three-year project to make tables with following variables:
| Initial Investment for purchase of machinery | $300,000 |
| Cash inflow in first year | $50,000 |
| Cash inflow in second year | $160,000 |
| Cash inflow in third year | $230,000 |
| Estimated scrap value of the machinery at the end of project | $40,000 |
| Cost of Capital of the company | 10% |
the NPV of the Project would be:
| Year/Cash flow | Year 0 | Year 1 | Year 2 | Year 3 |
| Initial Investment | ($300,000) | |||
| 1st cash inflow | $50,000 | |||
| 2nd cash inflow | $160,000 | |||
| 3rd cash inflow | $230,000 | |||
| Scrap Value | $40,000 | |||
| Net cash flows | ($300,000) | $50,000 | $160,000 | $270,000 |
| Discounting at cost of capital (10%) | × 1 | × 1/1.1 or 0.909 | × 1/1.12 or 0.826 | × 1/1.13 or 0.751 |
| Discounted Cash flows | ($300,000) | $45,450 | $132,160 | $202,770 |
| NPV | = -$300,000 + $45,450 + $132,160 + $202,770 = $80,380 | |||
At a 10% cost of capital, the net present value of this project is $80,380. Therefore it should be accepted by the Julia Private Limited.
To calculate IRR for this project, the NPV of the project is calculated using two different discount factors (i.e., two difference costs of capital) and then a rate of return at which NPV would be zero is estimated. We have already computed the NPV at 10% cost of capital. Now let’s compute a new NPV for the same project using a 20% cost of capital:
| Net cash flows | ($300,000) | $50,000 | $160,000 | $270,000 |
| Discounting at cost of capital (20%) | × 1 | × 1/1.2 or 0.833 | × 1/1.22 or 0.694 | × 1/1.23 or 0.579 |
| Discounted Cash flows | ($300,000) | $41,650 | $111,040 | $156,330 |
| NPV | = -$300,000 + $41,650 + $111,040 + $156,330 = $9,020 | |||
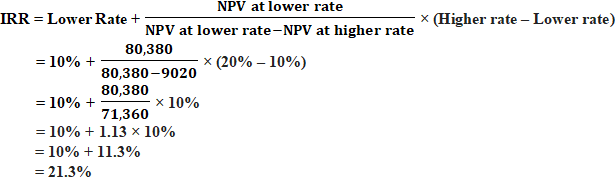
The project will be accepted as the internal rate of return (IRR) in above computations is greater than the cost of capital of 10%.
Difference between NPV and IRR:
The main difference between NPV and IRR is given below:
1. Outcome value:
The net present value (NPV) technique of investment appraisal shows the estimated net value of return in monetary terms that the project would generate. It considers the discounted value of all the possible cash outflows and inflows regarding a specific project and then compares the two to get a net positive or negative cash flow known as net present value.
The internal rate of return (IRR) method shows the value of return for a project in percentage terms. If IRR is applied to the cash flows of a project instead of cost of capital, the NPV of the project would be zero.
2. Basis of decision:
Generally, a project is accepted if its NPV is positive or it shows surplus funds at the end of the project. However, it is possible that a project generates positive cash flows but the business is still not ready to accept it because the positive NPV does not match the NPV set by the management of the business.
IRR is used to appraise the sensitivity of cost of capital which is used to appraise a project. IRR effectively shows a percentage below which NPV would start to fall negative. Therefore if IRR is greater than the cost of capital, the project is accepted and otherwise rejected.
3. Assumptions:
The NPV technique assumes that the cash inflows generated by the project are reinvested at the cost of capital of the business. This assumption is somehow realistic because the cost of capital of a business indicates the risk that a business is already facing in its investments.
The IRR method assumes that the cash inflows are reinvested at IRR or internal rate of return.
4. Cash flow variations:
The NPV calculation accommodates any cash outflows after the initial outflow of cash. If a later cash outflow occurs after the initial cash outflow, it can be discounted at applicable discount factor or cost of capital and included in the calculation easily.
The IRR calculation is disturbed by the cash outflows that occur after the initial cash outflow because in such a situation its calculations could produce more than one IRR’s which would be unrealistic. An alternative method of calculation available to overcome this problem is known as the modified internal rate of return (MIRR) method which is beyond the scope of this discussion.
5. Applications:
NPV enables a business to make constructive investment decisions because not only it accounts for whole project life but also takes into account the discounting factor which indicates the minimum amount of return the investors of business would agree upon or the level of return the business is already earning on its other investments.
IRR is used to indicate the risks attached to the project for which NPV is calculated. As the cash flows increase/decrease by an increase/decrease in the cost of capital of the company, IRR shows the percentage at which the project will be neither positive nor negative, which would indicate the sensitivity level of the cost of capital of that project.
NPV versus IRR – tabular comparison
A tabular comparison of NPV and IRR is given below:
|
||||
| Outcome Value | ||||
| In monetary terms. | In percentage terms. | |||
| Basis of Decision | ||||
| Project is accepted if NPV is positive. | Project is accepted if IRR is greater than the cost of capital. | |||
| Assumptions | ||||
| Cash flows are reinvested at the cost of capital. | Cash flows are reinvested at the IRR. | |||
| Cash outflow variations | ||||
| NPV calculation can accommodate for variable cash flows. | Simple IRR calculation method do not accommodate variable cash flows. Modified IRR or MIRR calculation can accommodate variable cash flows. | |||
| Applications | ||||
| Is used for appraising the outcome of a project. | Is used to measure the sensitivity of cost of capital of a company. | |||
Conclusion:
Investment appraisal is an integral part of the finance function of a business. Every business raises capital with the intention to invest it to earn profits. Investment appraisal aids the management of a company in making well-grounded investment decisions based on reasonable assumptions. NPV and IRR are both very effective appraisal tools, however these methods have their own limitations which must be considered with due care while relying on results generated by the application of these methods.
Accuracy of results of an NPV calculation are limited by the accuracy of variables used in calculations like life of the project, accuracy of cash flows, accuracy of cost of capital etc. Simple IRR method assumes a linear relationship between the NPV and rate of return of a project if plotted on a graph which is actually curved, but the method provides a reasonable estimate. Additionally, IRR cannot be used to compare two different investments because it may be the case that one investment has less IRR percentage but is generating more in cash terms than an investment having a higher percentage IRR but is generating less in terms of cash.
